Gears are not just used to transfer power, they also provide an opportunity to adjust the mechanical advantage of a mechanism. As discussed in the introduction to this unit, there are cases where a motor itself is powerful enough for an application but the motor’s output characteristics are not well suited to the application. A motor that is VERY fast but has only a little bit of torque would not be suitable to lift a heavy load; in these cases it is necessary to use gear ratios to change the outputs to a more appropriate balance of torque and speed.
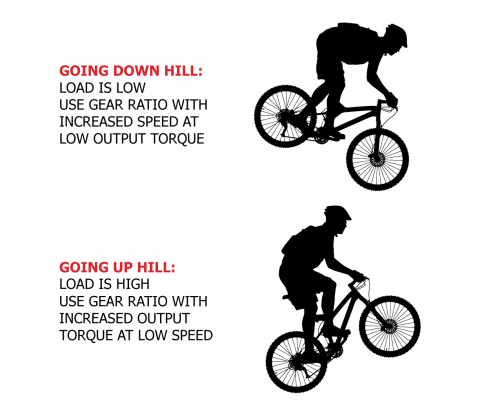
Think of a bicycle: the rider has limited power, and wants to ensure the power gets harnessed as much as possible at all times.
As the mechanical advantage changes, the speed of motion also changes. Power is the rate at which work is done. If the amount of work increases, the speed at which it gets done decreases.
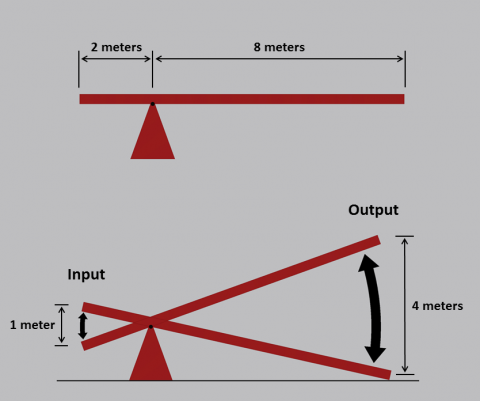
Example 8.1
In example 8.1, one can see that if the input side of the lever moves 1 meter then the output moves 4 meters. This difference is proportional to the ratio between the lengths of the levers. Thus, output length / input length = 8 / 2 = 4.
The interesting thing about this is that it moves these distances at the same time. Let’s say that it takes one second to move the input one meter, and the input is moving at one meter per second. At the same time, the output moves four meters in one second, and is moving at a speed of eight meters per second. The output is moving FASTER than the input, by the ratio of the lever lengths.
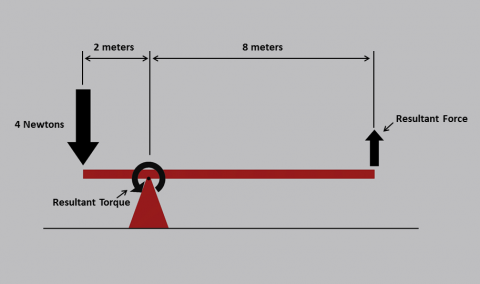
Example 8.2
In example 8.2, the same system shown in example 8.1 now has a 4 Newton force applied to the input. How much force then results at the output?
The first step is to calculate the applied torque on the center of rotation caused by the input force. Using the formulas from Unit 7:
Torque = Force x Distance from Center of Rotation = 4 N x 2 meter = 8 N-m
The second step is to calculate the resultant force that this torque now has on the output:
Force = Torque / Distance = 8 N-m / 8 meter = 1 Newton
So looking at the above two examples, if the lever system above has an input Force of 4 Newtons and moves 1 meter, the output will have a force of 1 Newton and moves 4 meters – it moves faster, with less force!
One can see how mechanical advantage (in the form of levers) can be used harness a fixed input force to accomplish a desired output. Gears work in the same manner.
A spur gear is basically a series of levers; the larger diameter the gear, the longer the levers.
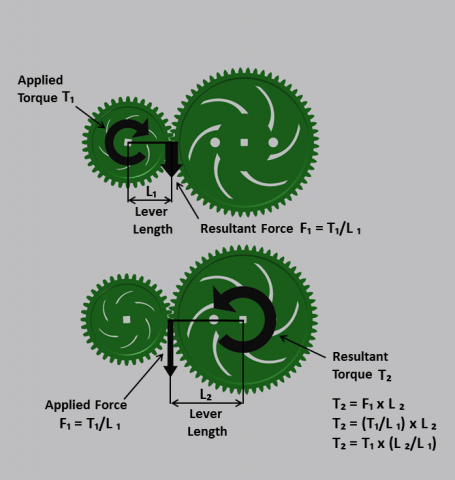
Example 8.3
As seen in example 8.3, torque applied to the first gear results in a linear force at the tip of the gear teeth. That same force is applied onto the tip of the tooth of the gear it is mated with, which in turn results in a torque rotating this gear. The diameters of the gears become the lengths of the levers, and the resulting change of torque is equivalent to the ratio of the diameters. Small gears driving large gears result in a torque increase. Large gears driving small gears result in a torque decrease.
Example 8.4
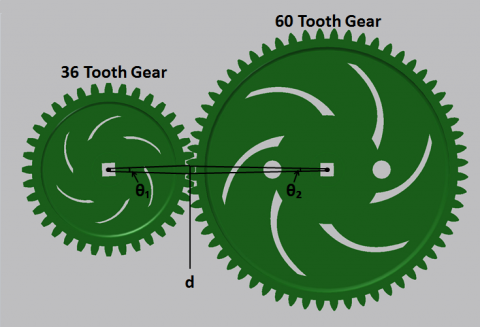
In example 8.4, if the input 36-tooth gear is rotated 1 tooth (d = 1 tooth width) thenthe gear is rotating 1/36th of a revolution (a1 = 360 / 36 = 10 degrees). As it advances it moves the 60-tooth gear 1 tooth also. However, on the 60 tooth gear this is only 1/60th of a revolution (a2 = 360 / 60 = 6 degrees).
As the small gear turns a certain amount in a given time the larger gear turns a smaller amount. This means the larger gear is spinning slower than the small gear. This concept works both ways. Small gears driving big gears result in a speed decrease. Large gears driving small gears result in a speed increase.
Combining the lessons of examples 8.1 through 8.4, one can see that the ratio between the sizes of two gears meshing is proportional to the resulting torque change and speed change between them. This is known as the Gear Reduction.
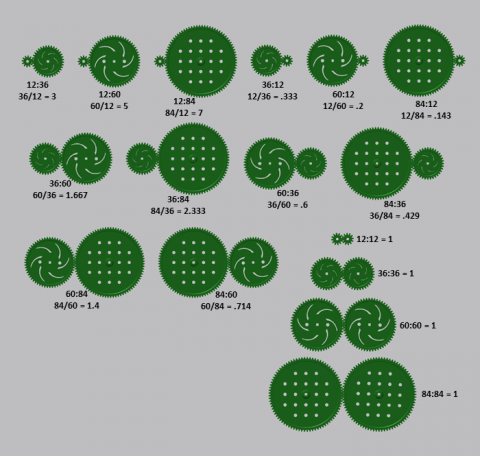
As discussed previously, the number of teeth a gear has is proportional to its diameter, so instead of using diameters to calculate Gear Reduction, one can just use tooth counts.
The Gear Ratio is denoted as (Driving Gear Teeth):(Driven Gear Teeth), so the above pair of gears could be described as 12:60 (or 36 to 60).
The Gear Reduction is calculated as Driven Gear Teeth / Driving Gear Teeth
So Gear Reduction = Driven Gear Teeth / Driving Gear Teeth = 60 / 36 = 1.67
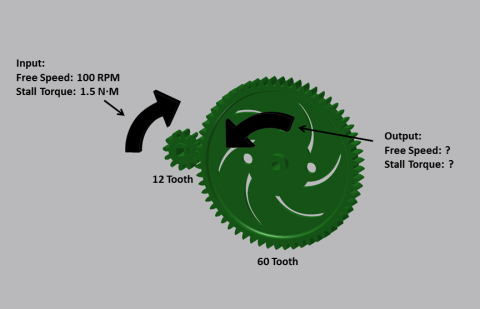
As discussed above, the Gear Ratio is denoted as (Driving Gear Teeth):(Driven Gear Teeth), so the above pair of gears could be described as 12:60 (or 12 to 60).
The Gear Reduction is calculated as Driven Gear Teeth / Driving Gear Teeth
So Gear Reduction = Driven Gear Teeth / Driving Gear Teeth = 60 / 12 = 5
Looking at the above example…
The stall-torque of the second shaft can be calculated using the following formula:
Output Torque = Input Torque x Gear Reduction
Output Torque = 1.5 N-m x 5 = 7.5 N-m
The free-speed of the second shaft can be calculated using the following formula:
Output Speed = Input Speed / Gear Reduction = 100 RPM / 5 = 20 RPM
So the secondary shaft spins with a free speed of 20 RPM and the stall torque is 7.5 N-m. The speed decreased, but the torque increased.
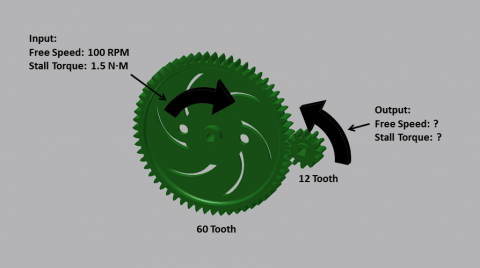
This second example can be calculated the same way.
Gear Reduction = Driven Gear Teeth / Driving Gear Teeth = 12 / 60 = 0.2
Output Torque = Input Torque x Gear Reduction = 1.5 N-m x 0.2 = 0.3 N-m
Output Speed = Input Speed / Gear Reduction = 100 RPM / 0.2 = 500 RPM
So the secondary shaft spins with a free speed of 500 RPM and the stall torque is 0.3 N-m. The speed increased, but the torque decreased.